Decoding a Warrant Name

Warrant Name
Underlying
Strike Price
Issuer
Warrant Type:  European or American
Warrant Class:  Call Warrant or Put Warrant
Expiry Date


Other Terms
Warrant Price
In the Money/At the Money/Out of the Money (Strike Price > or = or < Underlying Price)
Maturity: Short Term < 3 mths, Medium Term 3 to 6 mths, Long Term > 6 mths
Underlying Price
Days to Maturity
Implied Volatility
Interest Rates
Dividend
Historical Volatility
Conversion Ratio
Turnover
Outstanding Quantity
Premium:  positive, negative (discount), shrinking
Effective Gearing
Gearing
Delta
Vega, Gamma, Rho
Tick value
Face value
Bid/Ask Spread
Settlement Price

Stock Warrants:  Stock Put, Stock Call
Local Index Warrants:  Index Call Warrant, Index Put Warrant
Foreign Index Warrants:  Call or Put
Currency Warrants

Last Trading Day
Expiry Date
Payout Date

Option Pricing for Beginners

Posted on May 22, 2009 by James Kwak 

For a complete list of Beginners articles, see Financial Crisis for Beginners.

I’ve had two posts so far on the terms under which Treasury sold back to Old National the warrants on Old National stock that Treasury got in exchange for its TARP investment, so I thought it was time for an introduction to warrant/option pricing.

The warrants received by Treasury give Treasury the right to buy common stock in the issuing bank under predefined terms. Buying the stock is calledexercising the warrant. The warrant specifies how many shares Treasury can buy; the price that it must pay to buy them (the exercise price); and the term of the warrant, meaning how long Treasury has to decide whether or not it wants to exercise the warrant. If Treasury never exercises the warrant, then it expires and nothing happens. For our purposes, a warrant is the same as a call option; there are some differences I will ignore, which are outlined here.

Warrant terms

These warrants were part of the terms of the TARP Capital Purchase Program, which is what Treasury used to recapitalize banks last fall, starting in October. The warrants have value for Treasury – how much, I’ll get into later. Therefore, they make it possible for Treasury to be more generous with other terms of the transaction. Arguably, the warrants helped compensate for the fact that Treasury was buying preferred stock with a very low dividend yield – only 5%. There is no way that most banks would have been able to issue new preferred stock with only 5% dividends back in October-November. Probably the more important reason the warrants were mixed in was that they made it easier to justify the transaction politically; through the warrants, the taxpayer could “participate in the upside” if things went well, because if the stock price went up, the warrants would become more valuable.

As part of the Capital Purchase Program, banks had to give Treasury warrants on common stock equal in value to 15% of the amount of money invested. Treasury invested $100 million in Old National, so it needed warrants on $15 million worth of common stock. So it got warrants to buy 813,008 shares at an exercise price of $18.45; 813,008 * 18.45 = 15 million, or something very close to it. $18.45 represented the value of the common shares at the time of the investment. The idea is that the warrants were supposed to be “at the money;” if the stock went up, Treasury could exercise the warrants and make money; but if it went down, Treasury would get nothing (at least not from exercising the warrants). 

Actually, that isn’t quite accurate, for two reasons. First, according to the original term sheet, the exercise price was set not at the share price on the investment date itself, but as the average of the closing price for the twenty previous trading days; the idea here, which is common, is to protect both sides against day-to-day swings in stock prices. In Old National’s case, that would have been $16.35. However, in early April the Wall Street Journal reported that Treasury changed the terms to base the exercise price on the date that the bank’s application to participate in the CPP was approved, which was an earlier date. Because November-December was a period of falling bank stock prices, in the large majority of cases the change in dating had the effect of increasing the exercise price of the warrants, thereby reducing the value of the warrants to Treasury (because it would have to pay more for each share). In Old National’s case, it produced an exercise price of $18.45 instead of $16.35.

(Ilya Podolyako actually drafted a post about this at the time, but I chose not to publish it because I didn’t want to be hammering Treasury for every little thing they did that helped the banks. But I think it’s an important part of this story. Ilya also pointed out that when private companies do this kind of thing – setting the exercise price based on market prices in the past – it’s called backdating, and it’s illegal. My apologies to Ilya for not publishing the post then.)

Those warrants have a term of 10 years, meaning that Treasury has until 2018 to decide whether or not to exercise them. They also have an unusual “Reduction” feature, which says that if the bank raises more money than Treasury invested by the end of 2009, through sales of new common or perpetual preferred stock, half of the warrants will instantly evaporate. 

Warrant pricing

So how much are these things worth? On the date of the sale, Old National’s common shares were trading at $14.70 – $3.75 below the exercise price of the warrants. So if Treasury had done the crazy thing and exercised the warrants, it would have paid $18.45 for a share of stock worth $14.70, for a total loss of about $3 million.

However, the warrants themselves, like all options, always have some positive value, as long the term has not expired. You never have to exercise the warrants, so in no scenario will you be forced to lose money on them; and there is always some chance that the stock price will go above the exercise price, at which point you could exercise them and make money. The question is how much.

Conceptually speaking, you are trying to figure out the chances that the stock will someday be worth more than $18.45, times the profit you will make from exercising the warrants at that point. This clearly depends on the following parameters:

• Exercise price: The higher the exercise price, the less likely your warrant is to make you money.
• Current stock price: The higher the current price, the more likely you are to make money.
• Time to maturity: The more time you have, the higher the chances that the stock price will climb above the exercise price.

And it depends on one more parameter: volatility or, roughly speaking, the tendency of the stock price to move up and down. In the case of Old National, the stock price has to go up by $3.75 (25.5%) before the warrant can be exercised at a profit; the more volatile the stock, the more likely this is.

Making some additional assumptions, like zero transaction costs and zero dividends, Fischer Black and Myron Scholes worked out a formula to calculate the value of an option from these parameters (and the risk-free interest rate, since you are looking at the future and money loses value over time), which is now known as the Black-Scholes formula, and has been described as the central pillar, for better or worse, of modern finance. (Nassim Taleb strongly disagrees.) I think I had to derive the formula in a micro class a long time ago, but my memory of that year is a bit fuzzy, perhaps because I met my wife in that class.

In any case, the formula incorporates this useful intuition: To calculate the value of an option, you only need to know the expected value of exercise on the maturity date. This is because, theoretically, that is the only day on which you should ever exercise an option. Even if your option is $10 “in the money” (market price exceeds exercise price by $10), there is always a little bit of extra option value, because the potential upside is infinite, and the potential downside is bounded by $10. 

Note that the formula says you can price an option without even having an opinion about the fundamental value of the underlying stock – all you need are its current price and its volatility. This is consistent with a general (though not necessarily correct) principle that stock markets always efficiently price assets, so any opinion you may have about the stock’s fundamental value is foolish.

Also note that the key assumption in the formula is that stock prices will move randomly with constant volatility, and the key parameter in the formula is volatility. The other inputs are basically observable (though not quite in the case of the risk-free rate), but volatility is not. You need to know the volatility of the stock price between now and the maturity date, but all you can see is its volatility in the past. This makes option pricing especially difficult right now, because stock price volatility has been much higher over the last eight months than over the previous eight years. (The chart is the implied volatility of the S&P 500 since 2000.)

So if you use the volatility over the last eight months, you will get a much higher warrant value than if you use the volatility over the last eight years. More fundamentally, using any volatility assumption based on past data falls into the trap of assuming that the future will be like the past. This is never a foolproof assumption, and the longer the timeframe you are looking at, the worse the assumption becomes. It usually may not matter a lot for typical short-dated options (30 days, 60 days, etc.) – unless the world changes during those 30 days – but it matters a lot for long-dated warrants, like the 10-year warrants that Treasury got.

Real stocks also pay dividends, and the higher the future dividends, the less your warrant will be worth – because those dividends essentially come out of the future stock price. So your formula has to have some estimate of what dividend payouts will be. Again, this is especially hard right now, because many banks – including Old National – have drastically cut their dividends recently, and it’s difficult to predict when they will go back to paying higher dividends. 

Finally, the “Reduction” feature of the TARP warrants throws another wrench into the works. To value the warrants, you have to take into account the fact that half of them could vanish if Old National raises $100 million by issuing stock before the end of the year; and as long as the warrants were outstanding, they had an incentive to raise that money. That involves making guesses about the overall funding climate, and the corporate strategy of Old National, neither of which can be statistically estimated.

So now you should know enough to understand the three key assumptions behind the estimates in Linus Wilson’s paper. (However, the Bloomberg story does not provide its option pricing assumptions.) You should also be able to follow the discussion over assumptions between q and Sandrew in the comments to my previous post, beginning here.

What should Treasury have done?

q, a regular commenter here, concludes that the price Treasury got is within the range of reasonableness, given his preferred set of assumptions. However, he also says (agreeing with Nemo) that Treasury should not have negotiated a sale to Old National, but should have simply held onto them until maturity (remember, you don’t want to exercise them early); if the real issue was restrictions placed on TARP money, the government could have rolled them back (for banks that bought back their preferred shares). Or, if Treasury didn’t want to hold onto them, they could have auctioned them off.

While these are economically superior to simply negotiating a sale in a market with a single potential buyer (Old National), it gets us into the complicated world of TARP terms and conditions. First, the original term sheet said that Treasury could not sell more than 50% of the warrants before the end of 2009, because, remember, 50% of the warrants would vanish if the bank made a qualifying equity offering. Still, Treasury could have sold half and then held the rest; this would have had the salutary effect of giving Old National an incentive to raise new capital.

Second, assuming Treasury did not sell the warrants, when Old National bought back its preferred shares, it got the right to buy back the warrants at “fair market value” – but there is no market. (You can get a quote on short-dated options, but not long-dated ones – these are typically over-the-counter.) I haven’t found the implementation rules, but an article in Fortune said this:

February’s stimulus legislation – which gave TARP recipients the right to repay funds without raising new capital or observing any waiting period – specified that Treasury must liquidate a bank’s warrants at the current market price after it repays its TARP preferred stock.

I gather from bits and pieces I remember reading that there is some sort of appraisal process where the bank and Treasury first try to agree on a value, and I believe if that fails then there is supposed to be an auction. Auction participants would know all about option pricing, of course, and would apply a range of assumptions; presumably the sale would go to the buyer with the highest volatility assumption, which would probably (but not certainly)  yield a higher price than Treasury got. 

Of course, the banks have their opinion about all this (from the same Fortune article):

The American Bankers Association trade group last week sent Treasury Secretary Tim Geithner a letter calling for the government to eliminate the warrant-repayment provision altogether. The ABA said repurchasing the warrants amounts to an “onerous exit fee” for banks that have already repaid in full the funds they got from Treasury. . . .

Treasury must attempt to liquidate the warrant, the stimulus legislation says. But the ABA decries this as well, saying in its letter that selling the warrant to a third party could unfairly dilute a bank’s shareholders.

In other words, Treasury should just rip up the warrants – even though the warrants were one reason why the banks got investments on such generous terms in the first place. How times have changed since last fall.

By James Kwak

http://baselinescenario.com/2009/05/22/option-pricing-for-beginners/

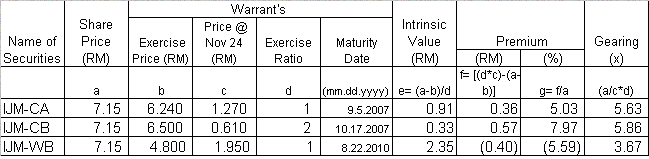
Warrants: Premium

Premium is a measure of how much the underlying price has to move for the warrant to break even if it is held until maturity.

The premium for a call warrant

= [Strike Price + (Warrant Price x Conversion Ratio) - Underlying Price] x 100% / Underlying Price


(1)  Cost of buying a warrant = Warrant Price x Conversion Ratio
(2)  Breakeven point of the warrant = Strike Price  + Cost of component
(3)  Premium = [(Breakeven point of warrant - Underlying Price ) / Underlying Price]  x 100%


In this formula, we first calculate the difference between the breakeven point and the underlying price and then divided it by the underlying price to find out the premium as a percentage.


Likewise, the premium for a put warrant 

= {Underlying Price - [Strike Price - (Warrant Price x Conversion Ratio)]} x 100% / Underlying Price


For example:

Company ABC Call Warrant currently trading at $0.54, with a strike price of $12 and a conversion ratio of 5:1.  If the underlying price is $14, how much is the premium?

Cost of buying the warrant = $0.54 x 5 = $2.70
Breakeven point = Strike Price + Cost = $12 + $2.70 = $14.70
Underlying price = $14.00
Difference between Underlying price and Breakeven point = $14.70 - $14,00 = $0.70
Premium = ($0.70/$14.00) x 100%  = 5%.

In other words, if the investor intends to hold the warrant until maturity, it takes a 5% increase in the underlying price from its current level of $14 to breakeven.

In this example, what we have is an out-of-the-money (OTM) warrant, and the underlying must make a bigger climb to reach the breakeven point.

In the case of an in-the-money (ITM) warrant, a modest increase in the underlying price would be enough.




Summary:

Step 1:   First, calculate the breakeven point of the warrant. This is done by using the formula:  [(price of warrant x conversion ratio) + strike price]

Step 2:  Work out the difference between the breakeven point and underlying price and divide this by the underlying price to get the premium in percentages.



The premium only measures the percentage increase in the underlying price that will allow the warrant investor to break even upon maturity.

It does not tell us whether the price of a warrant is too high or too low.

Hence, unless you are prepared to hold the warrant until maturity, premium is not a relevant indicator for you.

Warrants: Option Pricing Model

An example:

Warrants: Turnover versus Outstanding Quantity

Turnover is the total units of a warrant bought and sold on a day.

Outstanding quantity refers to the accumulated units, or the accumulated overnight positions, held by investors (other than the issuer) at the close of trading.

Outstanding percentage is the portion held by investors of the total units of the warrant in issue.



Various scenarios and interpretations of the market.

Day Trader >>> Overnight Traders  -  Turnover >>> Outstanding quantity

On a trading day when the market is dominated by day trade investors rather than overnight traders, the turnover can be way above the increase in outstanding quantity.


All new positions held overnight - Turnover = Increase in outstanding quantity 

In contrast, if all the new positions of the day are held overnight, the increase in outstanding quantity will be equal to the turnover.


Day trade market - High turnover + Flat outstanding quantity

Normally, when a high turnover meets a flat outstanding quantity, what we have is a day trade market.
This may be a sign of a lack of confidence in the outlook for the warrant.


Market dominated by sell orders - High turnover + Fall in outstanding quantity

When a high turnover meets a fall in outstanding quantity, then the market is dominated by sell orders.
This may mean that the holders of a call warrant are selling on expectation that the underlying is topping out (or bottoming up in the case of a put warrant).


Players are upbeat about the market outlook - High turnover + Increase in outstanding quantity

When a high turnover meets an increase in outstanding quantity, the investors here are probably long-term players who are rather upbeat about the market outlook.

Warrants: Historical Volatility

Historical volatility reflects the historical price of a stock within a given period of time.

If Stock A is trading at $10 with a volatility of 10%, then based on the theories of statistics, there is

-  68% of the time that the stock will be trading within the range of $9 to $11 ($10 +/- 1 S.D.),
-  95% of the time within the range of $8 to $12  ($10 +/- 2 S.D.)and
-  99.7% of the time within the range of $7 to $13  ($10 +/- 3 S.D.).

In other words, the higher the historical volatility of the underlying, the higher the level of its future volatility will be in a given period of time.


For the investors
Investors can use historical volatility to predict the future volatility and price direction in order to formulate their investment strategies.

For the issuer
For the issuer, historical volatility is one of the factors they need to take into account in determining the price of a warrant.
Where the historical volatility of its underlying is high, a warrant is likely to be issued at a higher price. However, past performance may not indicate future trends.
Hence, in the pricing process, an issuer will alos find out what the markte expects of the future volatility of the underlying, that is what we call the "implied volatility" of the warrant.

Warrants: Implied Volatility and Warrant Price

Apart from the underlying price, the most important factor that affects the price of a warrant is implied volatility.

It is the expected volatility of the underlying in a given future period of time and is positively related to the warrant price.

When the implied volatility of a warrant increases, its price may go up.

When the implied volatility decreases, the warrant price may go down.




An example:

Stock A is currently trading at $10.  The market expects that the range of fluctuations of the stock will be within $1 for most of the time in the future.

Stock B is currently trading at $10, and the market expects that its range of fluctuations will be within $5 for most of the time in the future.

What is the probability that stock A will climb to $20 within 6 months?

Which one, between Stock A and Stock B, will have a better chance of hitting $20 in 6 months?

Obviously, the answer is Stock B.


If for some reasons, the market expects a drop in the volatility of  stock B (say from $10 to $1 in terms of the range of fluctuations) in a given period of time, then the price of a related warrant may go down as well.

This is due to the lower probability that the price of Stock B will exceed the strike price of the warrant upon maturity.

Hence, there is less chance for the warrant to be exercised upon maturity, and the investor will also have a less chance to get a higher return.  As a result, the warrant price is likely to fall.

American Warrants versus European Warrants

Warrants can be divided into American or European types, based on the way they are exercised.

American Warrant - Holder can exercise the right to buy (or sell) the underlying at any time between the listing date and the expiry date.

European Warrant - Holder can exercise the same right only at maturity.



American Warrant

American Warrants can be exercised at any time between the listing date and the expiry date.

They seem to be more flexible.  However, in practice, few investors choose to exercise their warrants and hence, this feature does not matter much.

It is often more beneficial to sell the warrant back to the market before expiry rather than holding it until the date to exercise (the issue of "time decay").


European Warrant

European Warrants are settled by cash rather than physical delivery.

This means that if the warrants are in the money, the issuer will calculate and pay the difference between the settlement price of the underlying and the strike price of the warrant.

Cash settled warrants are automatically exercised, there is no need for the issuer to serve any notice of exercise.

Warrants: Conversion Ratio

The conversion ratio determines the number of warrants required for conversion into one share of the underlying stock or one point of the underlying index at maturity.

For example, where the conversion ratio is 10:1, 10 units of warrants will be required to be exchanged for each share of the underlying stock.

Even for warrants with identical terms (same strike price, maturity and implied volatility), their prices may vary hugely.

These warrants are worth exactly the same.  Their prices vary in proportion to the difference in their conversion ratios.

The price of one may be a few cents while the other a few dollars.  This is due to their conversion ratios.

The bigger the conversion ratio, the lower the warrant price.



Conversion Ratio is Insignificant as a performance indicator

Psychologically, investors tend to prefer warrants with a lower face value.

After all, warrants of different price ranges do differ in tick movement.

In theory, the difference in the conversion ratio will not affect the price performance of warrants.

When you are picking a warrant, do not be bothered with insignificant data such as the conversion ratio or premium.  

Unless you want to hold the warrant until maturity, these data should not be a matter of concern.

Rather, to make sure that you are picking the right choice, you should check out carefully the other terms of the warrant, such as implied volatility and effective gearing.

(In calculating the value at maturity and the effective gearing of a warrant at any time, the conversion ratio is always taken into account.)

Technical Parameters of Warrants: Vega, Gamma and Rho

Vega

Vega measures the rate of change in the warrant price for each point of movement of its implied volatility.

No matter it is a call warrant or a put warrant, vega is always positive, indicating that the warrant price and its implied volatility always move in the same direction.

Vega can be an absolute value or a percentage relative to the warrant price.


Gamma

Gamma measures the sensitivity of the delta of a warrant to the price movements of its underlying.

The higher the gamma, the bigger the change in delta will be in reaction to a movement in the underlying price.

Gamma = Rate of Change of Delta / Rate of Change of Underlying Price

No matter it is a call warrant or put warrant, gamma is always positive.



Rho

Rho measures the sensitivity of warrant price to changes in the market interest rate.

Call warrants have a positive rho, meaning that the price of a call warrant moves in the same direction as the market interest rate.

In contrast, put warrants have a negative rho, and this shows that the price of a put warrant moves in the opposite direction to the market interest rate.

Given that changes in interest rates tend to be limited in the short term, their effect on warrant prices is minimal.

Technical Parameters of Warrants: Theta

Theta, also called time decay, measures the rate of change in the price of a warrant as its maturity is running short while all other things being equal.

It can be expressed as an absolute value or a percentage relative to the warrant price (theta / warrant price).

Unless in some special circumstances, the value of theta is usually negative, reflecting the declining value of a warrant as time passes.

Depicted in a chart form, the slope of the curve of time value becomes steeper as the warrant gets closer to its maturity.

This shows that time decay accelerates as time passes.


Additional notes:

In percentage terms, time value has the biggest impact on out of the money (OTM) warrants.

The value of a warrant consists of intrinsic value and time value.

They vary in absolute and relative terms for warrants with different strike prices and maturity dates.

In the case of OTM warrants, their intrinsic values are negligible.

In other words, time value makes up most of their values.

Hence, they are more sensitive to the passage of time.

As for in the money (ITM) warrants, given that a large part of their value is made up of intrinsic value, they are less sensitive to the passage of time, and such sensitivity decreases as the maturity date gets nearer.

Warrants: Effective Gearing versus Gearing

The biggest appeal of warrant trading lies in the leverage effect.

Investors only need to invest a small sum to earn a potential return close to or even higher than that from directly investing in the underlying.



Gearing

Gearing only reflects how many times the underlying costs versus the warrant.  

Its calculation formula is:

Gearing = Underlying Price / (Warrant Price   x  Conversion Ratio)



Effective Gearing

However, the rate of increase/decrease in the warrant price relative to the underlying price is not the same as gearing.

To estimate the increase/decrease in the warrant price relative to the underlying price, we should look to the effective gearing.

Effective gearing reflects the relationship between changes in the warrant price and in the underlying price.

Its calculation formula is:

Effective Gearing = Gearing  x  Delta

For example, the effective gearing of a warrant is 10 times, then, other things being equal, for every 1% change in the underlying price, the warrant price will in theory move by 10%.

Put simply, delta measures how much, in theory, the warrant price will move for a $1 change in the underlying price.

When you invest in warrants, you should look to their effective gearing, not gearing, as a reference for their risk/return performance.

Warrants: Days to Maturity

Warrants can be classified accordingly to the length of their remaining days to maturity.

Short term warrant:  Warrant with less than 3 months to maturity
Medium term warrant:  Warrants with 3 to 6 months left to maturity
Long term warrant:  Warrants with more than 6 months running to maturity.


Whether it is long-term or short-term, ITM or OTM, a warrant is after all a leveraged investment instrument.
Be cautious in funds allocation and stop-loss arrangements.

Do not get carried away by the potential return without considering your risk tolerance.


For example:

A general investor may consider a medium-term warrant with around 3 months running to maturity and a strike price around 5% above or below the underlying price.

More aggressive investors may go for OTM warrants with a shorter maturity.

For conservative investors, they may choose ITM warrants with a longer maturity.



The warrant price tends to be positively related to the length of maturity.

In theory, the longer the maturity, the more room for changes in the underlying price will be.

Given the greater chance for the warrant to be exercised, the warrant price will tend to be higher.

No matter for call warrants or put warrants, the warrant price tends to be positively related to the length of maturity.

Besides, a warrant expiring in 6 months is less affected by time decay than one expiring in 3 months.

Warrants with a longer maturity will see their time values fall slower, while those with a shorter maturity will see their time values fall faster.

Deep in the money (deep ITM) and far out of the money (far OTM) warrants

If we take into account the extent of difference between the strike price and the underlying price, warrants can be further classified into:

ITM
deep ITM
OTM and
far OTM.

Generally, where there is a 15% or above difference between the strike price and the underlying price, a warrant will be considered far OTM or deep ITM.

However, this 15% mark is merely a rough idea, not an absolute threshold.

One must also look in the volatitlity of the underlying.

Some warrants may be considered deep ITM or far OTM even if the difference between strike price and the underlying price is only 10% or more.

Warrants - In the money, at the money and out of the money

A warrant is described as in-the-money (ITM), at-the-money (ATM) or out-of-the-money (OTM), depending on the relationship between its strike price and its underlying price.

A call warrant is OTM when its strike price is higher than its underlying price.

It is ITM, when its strike price is lower than its underlying price.

The situation is just the opposite for put warrants.

When its strike price is higher than its underlying price, a put warrant is ITM; and when its strike price is lower than its underlying price, it is OTM.

No matter it is a call or put, if the strike price is equal to the underlying price, the warrant is said to be ATM.


Summary

Call Warrant

ITM Strike Price < Underlying Price
ATM  Strike Price = Underlying Price
OTM  Strike Price > Underlying Price

Put Warrant

ITM   Strike Price > Underlying Price
ATM  Strike Price = Underlying Price
OTM  Strike Price < Underlying Price



Additional Notes

Call Warrant
An investor can buy a call warrant if he is optimistic about the outlook for its underlying.
When the underlying price does go above the strike price, in theory, the investor can exercise the warrant to buy the underlying at the strike price.
Then he can sell it in the market to earn the difference.
In practice, warrants are traded on a cash settlement basis, and investors will be paid the difference directly.

Put Warrant
In the case of a put warrant, an investor can go for it when he is pessimistic about the market outlook.
If the underlying price is lower than the strike price, in theory, the invstor can exercise the warrant and buy the underlying from the market for delivery to the issuer at the strike price to earn the difference.
In reality, investors will be paid the difference directly.
If it turns out that the underlying price is higher than the strike price, the investor will lose the cost of the warrant.

(The above assumes that the investor will hold the warrant until maturity.  Indeed, investors can also "buy low, sell high", and trade warrants just like stocks.)

Covered Warrants

Covered Warrants are mainly issued by investment banks.

They are issued to offer a leveraged investment tool for investors.

Cash settlement is the norm for Covered Warrants; thus companies will not face any changes in their shareholding structures as a result.

In other words, Covered Warrants will not dilute a company shareholding.


Unlike Company Warrants, Covered Warrants have good liquidity due to the market making system.

Their pricing mechanism is more transparent (statistics such as effective gearing is readily available).

It is possible to track changes in the theoretical prices of Covered Warrants.

Investors should study the relevant information carefully and bear in mind their own risk tolearance in making the decision whether to invest in Covered Warrants.

Penny Warrants are very risky.

Warrants with only 1 - 2 weeks left to maturity and over 10% out-of-the-money (OTM) are called penny warrants.

They are very risky and their odds are low.  The reasons are as follows:

1.  The bid/ask spreads of penny warrants are rather wide.

2.  Penny warrants have a very high rate of time decay.

3.  It is easy to lose money with penny warrants.

4.  Penny warrants may be not that price sensitive.  

5.  Penny warrants can hardly edge up but easily plummet.

Company Warrants

The basic concept of warrants is to give investors the right to buy or sell the underlying at the pre-determined strike price on the pre-determined date.

Company Warrants are issued by companies to raise funds or to reward employees or shareholders.

Upon maturity of a Company Warrant, provided that the stock price is higher than the strike price at the time, the holder is entitled to buy a certain number of shares of the company at the strike price.

When the holder does exercise the warrant, the company must issue new shares to meet the promise.

So, when Company Warrants are exercised, the shareholding of the company will be diluted.

Company Warrants normally have lower liquidity, and there is no way to compare their prices.

This is because the price of a Company Warrant is mainly determined by the board of directors.

Therefore, the warrant price is very likely to deviate from the underlying price.

Put another way, Company Warrants are less transparent and, sometimes, more speculative.  

Investors should study the relveant information carefully and bear in mind their own risk tolerance in making the decision whether to invest in Company Warrants.

Warrants versus Stocks

If you are optimistic about a stock, the most direct investment strategy is to buy the stock.

However, some investors may choose to buy a related warrant instead.


Pros:  Limited investment amount.

The biggest advantage of warrants is the leverage effect, which allows you to invest with less capital for the same return, as compared with stock trading.

The lower capital required for warrants means that, in case there is a market downturn, the loss will be limited as compared with investing directly in the stock.

In fact, in a number of major setbacks in the past, even giant blue chips fell sharply.  Buying warrants instead of stocks can help minimize one's exposure to such market risks.


Cons:  Time constraint

Of course, warrants are not without their shortcomings.

It takes a lot of time to understand the factors that may affect warrant prices before one can master the leverage effect to one's advantage.

Investors must get to know that warrants are subject to the time constraint.

The price of a warrant may change along with the implied volatility and dividend payout of its underlying and interest rates.

Even if you get the underlying direction right, you may still fail to reap the expected return.  

In case you get it wrong, you should stop the loss.

Never sit on your holdings like a stock investor does to wait for a rebound.  The price of a warrant will be dragged down by not only a falling underlying price, but also a declining time value.



Additional notes:

Warrants are derivatives.
They are an alternative investment to their underlying, and vice versa.
Warrants can never be an absolute substitute for their underlying.
Do manage your portfolio flexibly by investing in warrants and/or their underlying in light of the market conditions and outlook, as well as your own risk tolerance.
If one gets the market wrong, one will lose more from warrants (actual loss over investment cost) than from stocks.
That means the leverage effect of warrants is a double-edged sword.  (Investors must take caution.)
The investment cost for warrants is lower than that for stocks, but they are more volatile.
Hence, their rate of potential gain or loss is much higher than their underlying.

Summary:
Warrants are a leveraged investment tool.
Don't foreget that the leverage effect can mean more profit, but also more loss.
So do limit your investment amount in warrants.

Index Futures

In terms of trading, index futures are more straightforward.

When the index rises by a certain percentage, an index future buyer will gain while an index future seller will lose, exactly the same amount.

Trading in index futures is a zero sum game, and buyers and sellers gamble against each other.

Regarding capital requirement, a margin is payable upfront for an index future contract.

The investor has to pay the shortfall (margin call) to maintain the account balance at not less than the maintenance margin level.

An example:  The initial margin required for a particular Index futures contract is $688 and the maintenance margin required is $550.  Each point of the index is priced at $16.  The investor will face a margin call if the particular index drops by more than 13 points, as the investor has to pay the shortfall to maintain the account balance at not less than the maintenance margin level.