Is There An Opportunity With Johnson & Johnson's (NYSE:JNJ) 41% Undervaluation?

Comment:   

An example of using 2 stage growth model and discount cash flow method in valuing a company.

The discount cash flow method is based on 2 assumptions:   future cash flows and the applied discount rate.  

It is not an exact science.  One should you conservative assumptions in your valuation.

Charlie Munger mentioned that he had never seen Warren Buffett using the DCF method in his valuation.   There are better and easier ways to value a company.  Often you will know if a company is cheap or very expensive, even without having to do elaborate studies.   (An analogy is you do not need to know the weight to know that this person is overweight or obese or underweight.)  

Keep your valuation simple.  It is better to be approximately right than to be exactly wrong.

The article below shares how to do valuation in detail.

Happy investing.  

Mon, 4 March 2024 at 7:00 pm GMT+8·6-min read

Key Insights

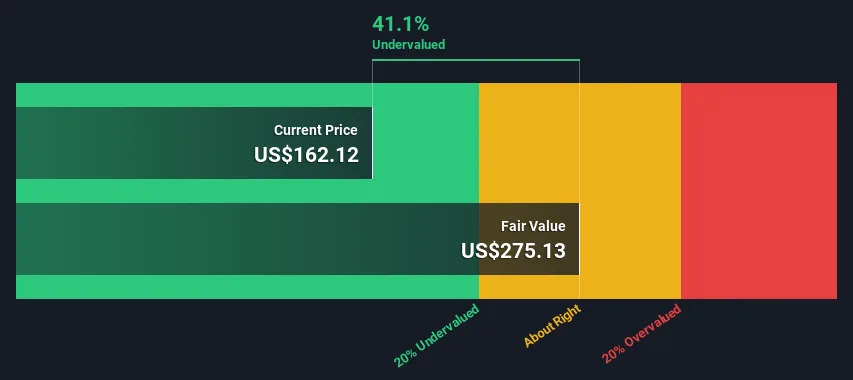
• Using the 2 Stage Free Cash Flow to Equity, Johnson & Johnson fair value estimate is US$275

• Johnson & Johnson is estimated to be 41% undervalued based on current share price of US$162

• Analyst price target for JNJ is US$174 which is 37% below our fair value estimate

Does the March share price for Johnson & Johnson (NYSE:JNJ) reflect what it's really worth? Today, we will estimate the stock's intrinsic value by taking the forecast future cash flows of the company and discounting them back to today's value. We will use the Discounted Cash Flow (DCF) model on this occasion. There's really not all that much to it, even though it might appear quite complex.

We generally believe that a company's value is the present value of all of the cash it will generate in the future. However, a DCF is just one valuation metric among many, and it is not without flaws. If you want to learn more about discounted cash flow, the rationale behind this calculation can be read in detail in the Simply Wall St analysis model.

Check out our latest analysis for Johnson & Johnson

What's The Estimated Valuation?

We're using the 2-stage growth model, which simply means we take in account two stages of company's growth. In the initial period the company may have a higher growth rate and the second stage is usually assumed to have a stable growth rate. To start off with, we need to estimate the next ten years of cash flows. Where possible we use analyst estimates, but when these aren't available we extrapolate the previous free cash flow (FCF) from the last estimate or reported value. We assume companies with shrinking free cash flow will slow their rate of shrinkage, and that companies with growing free cash flow will see their growth rate slow, over this period. We do this to reflect that growth tends to slow more in the early years than it does in later years.

A DCF is all about the idea that a dollar in the future is less valuable than a dollar today, so we discount the value of these future cash flows to their estimated value in today's dollars:

10-year free cash flow (FCF) estimate

	2024	2025	2026	2027	2028	2029	2030	2031	2032	2033
Levered FCF ($, Millions)	US$22.8b	US$23.9b	US$24.5b	US$25.0b	US$26.4b	US$27.2b	US$28.0b	US$28.7b	US$29.4b	US$30.2b
Growth Rate Estimate Source	Analyst x5	Analyst x6	Analyst x5	Analyst x3	Analyst x3	Est @ 2.99%	Est @ 2.78%	Est @ 2.63%	Est @ 2.53%	Est @ 2.46%
Present Value ($, Millions) Discounted @ 6.0%	US$21.5k	US$21.3k	US$20.6k	US$19.9k	US$19.8k	US$19.2k	US$18.6k	US$18.1k	US$17.5k	US$16.9k

("Est" = FCF growth rate estimated by Simply Wall St)
Present Value of 10-year Cash Flow (PVCF) = US$193b

We now need to calculate the Terminal Value, which accounts for all the future cash flows after this ten year period. For a number of reasons a very conservative growth rate is used that cannot exceed that of a country's GDP growth. In this case we have used the 5-year average of the 10-year government bond yield (2.3%) to estimate future growth. In the same way as with the 10-year 'growth' period, we discount future cash flows to today's value, using a cost of equity of 6.0%.

Terminal Value (TV)= FCF2033 × (1 + g) ÷ (r – g) = US$30b× (1 + 2.3%) ÷ (6.0%– 2.3%) = US$838b

Present Value of Terminal Value (PVTV)= TV / (1 + r)10= US$838b÷ ( 1 + 6.0%)10= US$469b

The total value, or equity value, is then the sum of the present value of the future cash flows, which in this case is US$663b. To get the intrinsic value per share, we divide this by the total number of shares outstanding. Relative to the current share price of US$162, the company appears quite good value at a 41% discount to where the stock price trades currently. The assumptions in any calculation have a big impact on the valuation, so it is better to view this as a rough estimate, not precise down to the last cent.

dcf

The Assumptions

The calculation above is very dependent on two assumptions. The first is the discount rate and the other is the cash flows. If you don't agree with these result, have a go at the calculation yourself and play with the assumptions. The DCF also does not consider the possible cyclicality of an industry, or a company's future capital requirements, so it does not give a full picture of a company's potential performance. Given that we are looking at Johnson & Johnson as potential shareholders, the cost of equity is used as the discount rate, rather than the cost of capital (or weighted average cost of capital, WACC) which accounts for debt. In this calculation we've used 6.0%, which is based on a levered beta of 0.800. Beta is a measure of a stock's volatility, compared to the market as a whole. We get our beta from the industry average beta of globally comparable companies, with an imposed limit between 0.8 and 2.0, which is a reasonable range for a stable business.

SWOT Analysis for Johnson & Johnson

Strength

• Debt is not viewed as a risk.

• Dividends are covered by earnings and cash flows.

Weakness

• Earnings declined over the past year.

• Dividend is low compared to the top 25% of dividend payers in the Pharmaceuticals market.

Opportunity

• Annual earnings are forecast to grow for the next 3 years.

• Good value based on P/E ratio and estimated fair value.

Threat

• Annual earnings are forecast to grow slower than the American market.

Next Steps:

Valuation is only one side of the coin in terms of building your investment thesis, and it shouldn't be the only metric you look at when researching a company. The DCF model is not a perfect stock valuation tool. Preferably you'd apply different cases and assumptions and see how they would impact the company's valuation. For instance, if the terminal value growth rate is adjusted slightly, it can dramatically alter the overall result. What is the reason for the share price sitting below the intrinsic value? For Johnson & Johnson, there are three pertinent aspects you should consider:

1. Risks: Every company has them, and we've spotted 1 warning sign for Johnson & Johnson you should know about.

2. Future Earnings: How does JNJ's growth rate compare to its peers and the wider market? Dig deeper into the analyst consensus number for the upcoming years by interacting with our free analyst growth expectation chart.

3. Other Solid Businesses: Low debt, high returns on equity and good past performance are fundamental to a strong business. Why not explore our interactive list of stocks with solid business fundamentals to see if there are other companies you may not have considered!

PS. The Simply Wall St app conducts a discounted cash flow valuation for every stock on the NYSE every day. If you want to find the calculation for other stocks just search here.

 editorial-team@simplywallst.com (Simply Wall St)

https://uk.finance.yahoo.com/news/opportunity-johnson-johnsons-nyse-jnj-110049724.html

Valuation of Common Stock with Temporary Supernormal Growth

The correct valuation model to value such "supernormal growth" companies is the multi-stage dividend discount model that combines

• the multi-period and 
• infinite-period dividend discount models (Gordon Growth Model).

Value
= Multi-period DDM + Infinite Period (constant growth) DDM
= D1/(1+k)^1 + D2/(1+k)^2 + ..... + Dn/(1+k)^n + Pn/(1+k)^n


Dn = Last dividend of the supernormal growth period
Dn+1 = First dividend of the constant growth period.
Pn = Dn+1 / (k-g) = PV at time n+1 of Dn at a Constant rate of Growth.

Applying Present Value Models

1.  Where Gordon Growth Model is highly appropriate

The Gordon Growth Model is highly appropriate for valuing dividend-paying stocks that are relatively immune to the business cycle and are relatively mature (e.g., utilities).

It is also useful for valuing companies that have historically been raising their dividends at a stable rate.



2.  Where DDM or Gordon Growth Model is difficult to use

Applying the DDM is relatively difficult if the company is not currently paying out a dividend.  

A company may not pay out a dividend because:

• It has a lot of lucrative investment opportunities available and it wants to retain profits to reinvest them in the business.
• It does not have sufficient excess cash flow to pay out a dividend.

Even though the Gordon Growth Model can be used for valuing such companies, the forecasts used are generally quite uncertain.

Therefore, analysts use one of the other valuation models to value such companies and may use the DDM model as a supplement.

3.  Multi-stage DDM can be employed 

The DDM can be extended to numerous stages.  For example:

A.   A three-stage DDM is used to value fairly young companies that are just entering the growth phase.  Their development falls into three stages - 

• growth (with very high growth rates), 
• transition (with decent growth rates) and 
• maturity (with a lower growth into perpetuity).

B.  A two-stage DDM can be used to value a company 

• currently undergoing moderate growth, but 
• whose growth rate is expected to improve (rise) to its long term growth rate.

Return on Share Investment = Dividend Yield + Growth over Time (Gordon Growth Model)

Rearranging the Dividend Discount Model (DDM) formula:

PV = D1 / (k-g)

k = (D1/PV) + g
   = Dividend yield + growth over time.

This expression for the cost of equity (required rate of return) tells us that the return on an equity investment has two components:

• The dividend yield (D1/PV at year 0)
• Growth over time (g)

Return on share investment = Dividend Yield + Growth over Time:

Dividend Discount Model

Dividend Discount Model

Where:

V = the value

D₁ = the dividend next period

r = the required rate of return

1.  One year holding period

If our holding period is just one year, the value that we will place on the stock today is the present value of the dividends that we will receive over the year plus the present value of the price that we expect to sell the stock for at the end of the holding period.

Present Value of the dividends that we will receive over one year 
= Dividend to be received/(1+r)^1

Present value of the price we expect to sell the stock for at the end of the holding period
= Year-end price / (1+k)^1


Value 
= PV of dividends receive over 1 year + PV of price we expect to sell at end of 1 year
= [Dividend to be received/(1+k)^1]  +  [Year-end price /(1+k)^1]

k = cost of equity or required rate of return



2.  Multiple-Year Holding Period DDM

We apply the same discounting principles for valuing common stock over multiple holding periods.

In order to estimate the intrinsic value of the stock, we first estimate the dividends that will be received every year that the stock is held and the price that the stock will sell for at the end of the holding period.

Then we simply discount these expected cash flows at the cost of equity (required return).

PV of Dividends received in Year 1 = D1/(1+k)^1
PV of Dividends received in Year 2 = D2/(1+k)^2
PV of Dividends received in Year .. =
PV of Dividends received in Year n= Dn/(1+k)^n
Price of stock sold at end of holding period n = Pn / (1+k)^n

Value
= PV of D1 + PV of D2 + PV of D3 +.................. PV of Dn + PV of Holding-Period Price
= [D1/(1+k)^1]  + [D2/(1+k)^2]  + .[D3/(1+k)^3]..........[.Dn/(1+k)^n]  + [Pn / (1+k)^n]




3.  Infinite Period DDM (Gordon Growth Model)

Assumptions of the Infinite Period DDM (Gordon Growth Model):

• The infinite period dividend discount model assumes that a company will continue to pay dividends for an infinite number of periods.
• It also assumes that the dividend stream will grow at a constant rate (g) over the infinite period.

In this case, the intrinsic value of the stock is calculated as:

Value = PV of D1 + PV of D2 + PV of D3 + ...........PV of Dn....... + PV of Dinfinity


PV of Dividends received in Year 1 = D1/(1+k)^1 = D0(1+g)^1/(1+k)^1
PV of Dividends received in Year 2 = D2/(1+k)^2 = D0(1+g)^2/(1+k)^2
PV of Dividends received in Year .. =
PV of Dividends received in Year n= Dn/(1+k)^n = DO(1+g)^infinity / (1+k)^infinity

D0 = Dividends received at year 0

This equation simplifies to:

PV at year 0
= D0(1+g)^1/(k-g)^1
= D1/(k-g)






The critical relationship between k and g in the infinite-period DDM (Gordon Growth Model)

The relation between k and g is critical:

• As (k-g) increases, the intrinsic value of the stock falls.
• As (k-g) narrows, the intrinsic value of the stock rises.
• Small changes in either k or g, can cause large changes in the value of the stock.

For the infinite-period DDM model to work, the following assumptions must hold:

• Dividend grows at a rate, g, which is not expected to change (constant growth).
• k must be greater than g; otherwise the model breaks down because of the denominator being negative.

(k-g) = difference between k and g or difference between cost of equity or required rate of return and growth rate.







Additional notes:

Return on investment = Dividend Yield + Growth over Time:

Rearranging the DDM formula:

PV = D1 / (k-g)

k = (D1/PV) + g
   = Dividend yield + growth over time.

This expression for the cost of equity (required rate of return) tells us that the return on an equity investment has two components:

• The dividend yield (D1/PV at year 0)
• Growth over time (g)

Multiples based on Fundamentals - the Justified P/E ratio

A price multiple may be related to fundamentals through a dividend discount model such as the Gordon growth model.

The expressions developed in such an exercise are interpreted as the justified (or based on fundamental) values for a multiple.


The justified P/E Ratio:

P/E = D/E/(r-g)

r = required rate of return
g = growth


Gordon Growth Model

r = D/P + g
D/P = r-g
P= D/(r-g)
P/E = D/E(r-g)
P/E = Dividend Payout Ratio / (r-g)



Inference

The P/E ratio is inversely related to the required rate of return.
The P/E ratio is positively related to the growth rate.
The P/E ratio appears to be positively related to the dividend payout ratio.

• However, this relationship may not always hold because a higher dividend payout ratio implies that the company's earnings retention ratio is lower.  
• A lower earnings retention ratio translates into a lower growth rate.  
• This is known as the "dividend displacement" of earnings.

Notes:

Higher the growth rate (g), higher the P/E.
Higher the required rate of return (r), lower the P/E.
Higher the DPO ratio, higher the P/E.
Also, higher the DPO ratio, lower the retained earnings,  leading to lower growth rate (g), thus lower P/E. ("dividend displacement" of earnings)